· Show that the function f(x) = 3x – 5 is a bijective function from R to R Solution Given Function f(x) = 3x – 5 To prove The function is bijective According to the definition of the bijection, the given function should be both injective and surjectiveBijective A function f (from set A to B) is bijective if, for every y in B, there is exactly one x in A such that f(x) = y Alternatively, f is bijective if it is a onetoone correspondence between those sets, in other words both injective and surjectiveAnswer f (x) = 1x2x2 =1− 1x21 Clearly domain of f (x) is R and range is 0,1) also f (−1) = f (1) Hence this function is neither injective not surjective
How To Prove The Rational Function F X 1 X 2 Is Surjective Onto Using The Definition Youtube
F(x)=x^2+1 is bijective
F(x)=x^2+1 is bijective-Taking the derivative of f (x) we get f' (x) = (1 x^ {2} 2x^ {2})/ (1x^ {2})^ {2}= (1x^ {2})/ (1x^ {2})^ {2} = since for all real x, 1 x^ {2} is positive and also the denominator is also positive this implies derivative is increasing also since the function is continous at x= 1 , f (x) tends infinityDemonstrating that $f(x) = x^2 1$ is bijective and calculating $f \circ f^{1}(x)$




Notes On Topics Of Algebra Notes
28/1/ · Putting f(x1) = f(x2) we have to prove x1 = x2 Since if f (x1) = f (x2) , then x1 = x2 ∴ It is oneone (injective) Check onto (surjective) f(x) = x3 Let f(x) = y , such that y ∈ Z x3 = y x = 𝑦^(1/3) Here y is an integer ie y ∈ Z Let y = 2 x = 𝑦^(1/3) = 2^(1/3) So, x is not an integer ∴ f is not onto (not surjective) Hence, function f is injective but not surjectiveAbout Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us CreatorsFunctions can be injections (onetoone functions), surjections (onto functions) or bijections (both onetoone and onto) Informally, an injection has each output mapped to by at most one input, a surjection includes the entire possible range in the output, and a bijection has both conditions be true This concept allows for comparisons between cardinalities of sets, in proofs comparing the
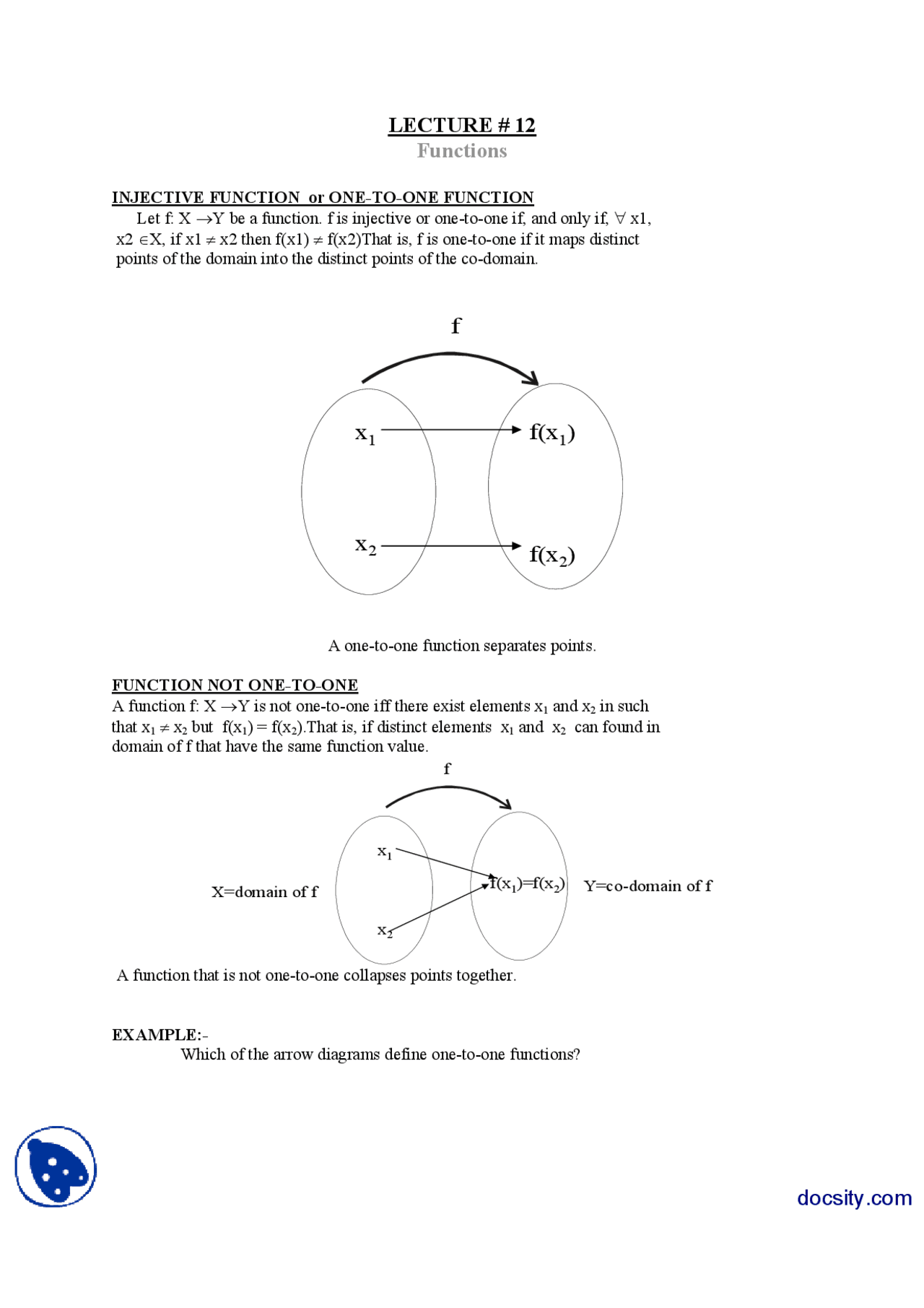
The function f is injective or onetoone if every point in the image comes from exactly one elementinthedomainToshowafunctionisinjectiveprove x 1;x 2 2A and f„x 128/1/ · Ex 13, 6 Show that f −1, 1 → R, given by f(x) = 𝑥/(𝑥 2) is oneone Find the inverse of the function f −1, 1 → Range f (Hint For y ∈ Range · An example of a function that is not injective is f(x) = x 2 if we take as domain all real numbers If we fill in 2 and 2 both give the same output, namely 4 So x 2 is not injective and therefore also not bijective and hence it won't have an inverse A function is surjective if every possible number in the range is reached, so in our case if every real number can be reached
We have function f(x) = 3 − 4x which is defined on R Let x 1, x 2 ∈ R such that x 1 ≠ x 2 ⇒ −4x 1 ≠ −4x 2 (Multiplying both sides by 4) ⇒ 3 − 4x 1 ≠ 3 − 4x 2 (Adding 3 both sides) ⇒ f(x 1) ≠ f(x 2) This implies that each different element having different images under the function f(x)Minimum f = 463, nearly This is improved to 8sd, \displaystyle {} , using an iterative numerical method15/9/ · Thus, f is not bijective Option B f (x) = x 2 Let f(x 1) = f(x 2) ⇒ x 1 2 = x 2 2 ⇒ x 1 = x 2 ⇒ f is one one Let f(x) = y, y ∈ Z ⇒ y = x 2 ⇒ x = y – 2 ⇒ for each y ∈ Z there exists x ∈ Z (domain) such that f(x) = y ⇒ f is onto Thus, f is bijective Option C f (x) = 2x 1 Let f(x 1) = f(x 2) ⇒ 2x 1 1 = 2x 2 1 ⇒ x 1 = x 2 ⇒ f is one one Let f(x) = y, y ∈ Z ⇒ y = 2x 1 ⇒ y 1 = 2x




Show That The Function F R R Defined As F X X 2 Is Neit
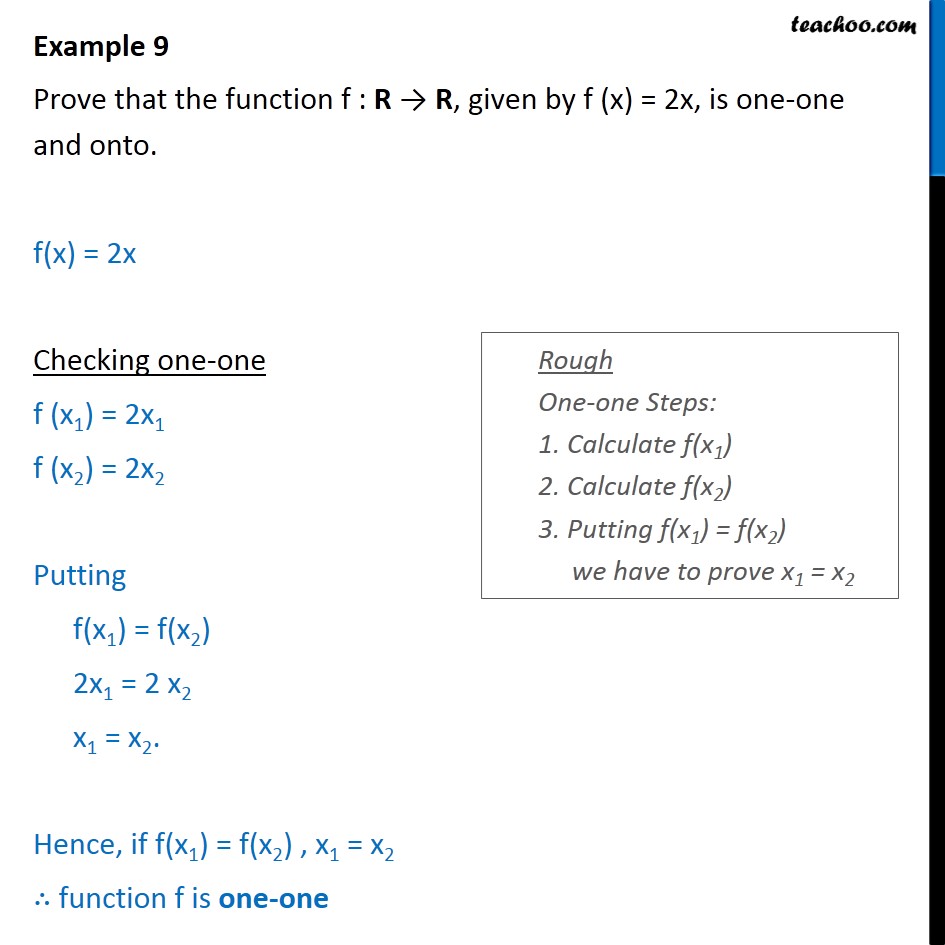



Example 9 Prove F X 2x Is One One And Onto To Prove One One O
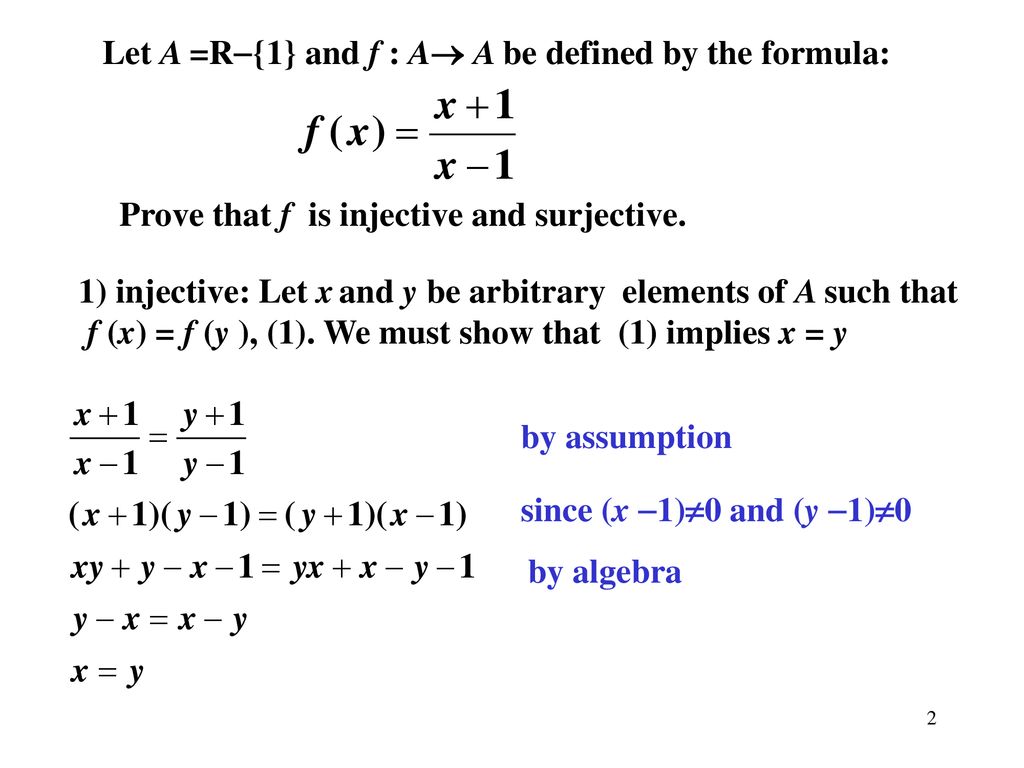
F(x) = 1/x is both injective (onetoone) as well as surjective (onto) f R to R f(x)=1/x , f(y)=1/y f(x) = f(y) 1/x = 1/y x=y Therefore 1/x is one to one function that is injective Let f(x)=y 1/x = y x = 1/y which is true in Real number18/5/18 · Let A = R {3} and B = R {1} Consider the function fA→B defined by f(x)=(x2/x3) Show that f is oneone and onto and hence find f 1Let A = R {3 }, B = R {1 } If fA→ B be defined by f (x) = x 2x 3∀ x∈ A Then show that f is bijective




Ncert Exemplar Solutions For Class 12 Maths Chapter 1 Relations And Functions Avail Free Pdf




Tutorial 4 Questions Computational Mathematics Kqi7004 Studocu
To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW If `2f (x1)f((1x)/x)=x , then f(x)` isIf F(x)=x has no real solution then also F(F(x)=x has no real solutionFor every function f, subset X of the domain and subset Y of the codomain, X ⊂ f −1 (f(X)) and f(f −1 (Y)) ⊂ Y If f is injective, then X = f −1 (f(X)), and if f is surjective, then f(f −1 (Y)) = Y For every function h X → Y, one can define a surjection H X → h(X) x → h(x) and an injection I h(X




Solved Let F X In X Squareroot X 2 1 Find The Doma Chegg Com




Let F R Rightarrow R Be A Function Defined By F X X X N Where Represents Fractional Part Function N 1 Injective N 2 Surjective N 3 Bijective N 4 Not Bijective
The function f R → R, f(x) = 2x 1 is bijective, since for each y there is a unique x = (y − 1)/2 such that f(x) = y More generally, any linear function over the reals, f R → R , f ( x ) = ax b (where a is nonzero) is a bijectionLet A = R − (2) and B = R − (1) If f A B is a function defined by`"f(x)"=("x"1)/("x"2),` how that f is oneone and onto Hence, find f −1Answer ∴ f is an oneone function Then, y =1 The function f is onto if there x ∈ A such that f (x) = y ∴ f is onto Since f is one=one and onto then, the given function is bijective




The Function F R 1 2 1 2 Defined As F X X 1 X 2 Is



Classify Each Function As Injective Surjective Chegg Com
Find the Domain and Range f (x)=x^21 f (x) = x2 1 f ( x) = x 2 1 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval NotationSolve for x x = (y 1) /2 Here, y is a real number When we subtract 1 from a real number and the result is divided by 2, again it is a real number For every real number of y, there is a real number x So, range of f(x) is equal to codomain It is onto function Hence it is bijective function (ii) f R > R defined by f (x) = 3 – 4x 2 Solution8/11/18 · Let f A → B be defined by f (x) = x – 2/ x – 3 ∀ x ∈ A Then show that f is bijective asked Sep 15, in Sets, Relations and Functions by Chandan01 ( 512k points)




How To Determine If A Function Is One To One Mathematics Stack Exchange




You Know From Calculus That Functions Play A Fundamental Role In Mathematics Pdf Free Download
Example 1 The function f (x) = x 2 from the set of positive real numbers to positive real numbers is injective as well as surjective Thus, it is also bijective However, the same function from the set of all real numbers R is not bijective since we also have the possibilities f (2)=4 and f (2)=4Steps Using Derivative Rule for Sum f ( x ) = 3 x ^ { 2 } 1 f ( x) = 3 x 2 1 The derivative of a polynomial is the sum of the derivatives of its terms The derivative of a constant term is 0 The derivative of ax^ {n} is nax^ {n1} The derivative of a polynomial is the sum ofHence, the function f(x) is surjective Hence, since the function is both injective and surjective over the given domain and range, then the function is a bijection Approved by eNotes Editorial Team



Prove If G Circ F Is Bijective Is F Bijective Mathematics Stack Exchange




1 A Function F Z Zx Z Is Defined As F N 2n 1 Chegg Com
28/1/ · Ex 12 , 7 In each of the following cases, state whether the function is oneone, onto or bijective Justify your answer f R → R defined by f(x) = 3 − 4x f(x) = 3 – 4x Checking oneone f (x1) = 3 – 4x1 f (x2) = 3 – 4x2 Putting f(x1) = f(x2) 3 – 4x1 = 3 – 4x2 Rough OneoneThe function f is defined by f (x) = x4 −4x2 x 1 for −5 ≤ x ≤ 5 What is the interval in which the minimum of value of f Purely a graphical approximation;The Function F X 1 X Sinx Cosx 0 X Pi 2 The Function F X 2x 3 3x 2 90x 174 Is The Interval The Function F X Cot 1 X X Increases In The Interval The Function F X Log 1 X 1 X Satisfies The Equation The Function F X Px Q R X X Belongs To Infinity Where P 0 Q 0 R 0 The Function F X X 1 X Has The Function F X X 2 E X Increases In The Interval




F R R Given By F X X Sqrt X 2 Is A Injective B Surj




Are Even Functions Injective Mathematics Stack Exchange
Click here👆to get an answer to your question ️ Prove that the function FN→ N , defined by f(x) = x^2 x 1 is one one but not onto28/1/ · Ex 12, 1 Show that the function f R* → R* defined by f(x) = 1/x is oneone and onto, where R* is the set of all nonzero real numbers Is the result true, if the domain R* is replaced by N with codomain being same as R*?Now, f (x 1 ) − f (x 2 ) ⇒ x 1 3 If the mapping f A → B and g B → C are both bijective, then show that the mapping g o f A → C is also bijective Medium View solution




Question Bank Set Theory Function Mathematics Set Mathematics




Ex 1 2 2 Check Injectivity And Surjectivity Of I F X X 2
Graph f(x)=(x^21)/(x1) Rewrite the function as an equation Use the slopeintercept form to find the slope and yintercept Tap for more steps The slopeintercept form is , where is the slope and is the yintercept Find the values of and using the formWe take the old exponents and subtract one, so it's gonna give us a negative three, which means that we end up getting negative two x to the negative three Now, another thing is we want to evaluate it at a so f prime of a is just going to be a negative to a to the negative three And this will be our final answer for this problem Add To PlaylistA function is injective if and only if X1 = X2 implies f (X1)=f (X2) vice versa f X to 2X, it is obviously to prove X1 = X2 if and only if f (X1)=f (X2), injective or 1–1 Since any elements in form of 2X are from scalar multiplication with 2 by preimage "X", for all X



Check The Injectivity And Surjectivity Of The Following Functions I F N N Given By Youtube




State Which Of The Following Are One One And Onto Function F R Gtr Defined By F X 2x 1 Youtube
Then add the square of 1 to both sides of the equation This step makes the left hand side of the equation a perfect square x^ {2}2x1=4 Add 3 to 1 \left (x1\right)^ {2}=4 Factor x^ {2}2x1 In general, when x^ {2}bxc is a perfect square, it can always be factored asFind f f"(x) = 1/x^2 🚨 Hurry, space in our FREE summer bootcamps is running out 🚨Solving for f R* → R* f(x) = 1/x Checking oneone




If A Function F R To R Is Defined As F X X 2 1 Then Youtube




Ex 1 2 2 Check Injectivity And Surjectivity Of I F X X 2
28/1/ · Ex 13, 8 Consider f R → 4, ∞) given by f(x) = x2 4 Show that f is invertible with the inverse f−1 of given f by f1 (y) = √(y−4) , where R is the set of all nonnegative real numbers f(x) = x2 4 f is invertible if f is oneone and onto Checking oneone f (x1) = (x1)2 4 f (x11/2/ · Best answer Given f N → N, defined by f (x) = x2 x 1 Let us prove that given function is oneone Injectivity Let x and y be any two elements in domain (N), such that f (x) = f (y) ⇒ x2 x 1 = y2 y 1 ⇒ (x2 – y2) (x – y) = 0 ` ⇒ (x y) (x y) (x – y) = 0 ⇒ (x – y) (x y 123/8/19 · Explanation − We have to prove this function is both injective and surjective If f ( x 1) = f ( x 2), then 2 x 1 – 3 = 2 x 2 – 3 and it implies that x 1 = x 2 Hence, f is injective So, x = ( y 5) / 3 which belongs to R and f ( x) = y Hence, f is surjective Since f is both surjective and injective, we can say f is bijective




B F R R Defined By F X Solution The Function F Chegg Com




Notes On Topics Of Algebra Notes




The Function F R R Defined By F X 4 X 4 X Is




Slides Show



Functions Discrete Mathematics Lecture Handout Docsity




Relation And Function Ppt Download




Notes On Topics Of Algebra Notes




Prove That The Function F N N Defined By F X X 2 X 1 I




Determine The Function F R R Define By F X X If X Gt 2 5x 2 If X 2 Is A Injection Or Surjection Brainly In



Today S Topics Proof That A Given Function Is Injective Or Surjective Composite Functions Properties Of The Composite Functions Inverse Functions Proofs Ppt Download



Surjective Onto And Injective One To One Functions Video Khan Academy




Question A1 A Bijective Function F 0 8 To 0 8 Gauthmath
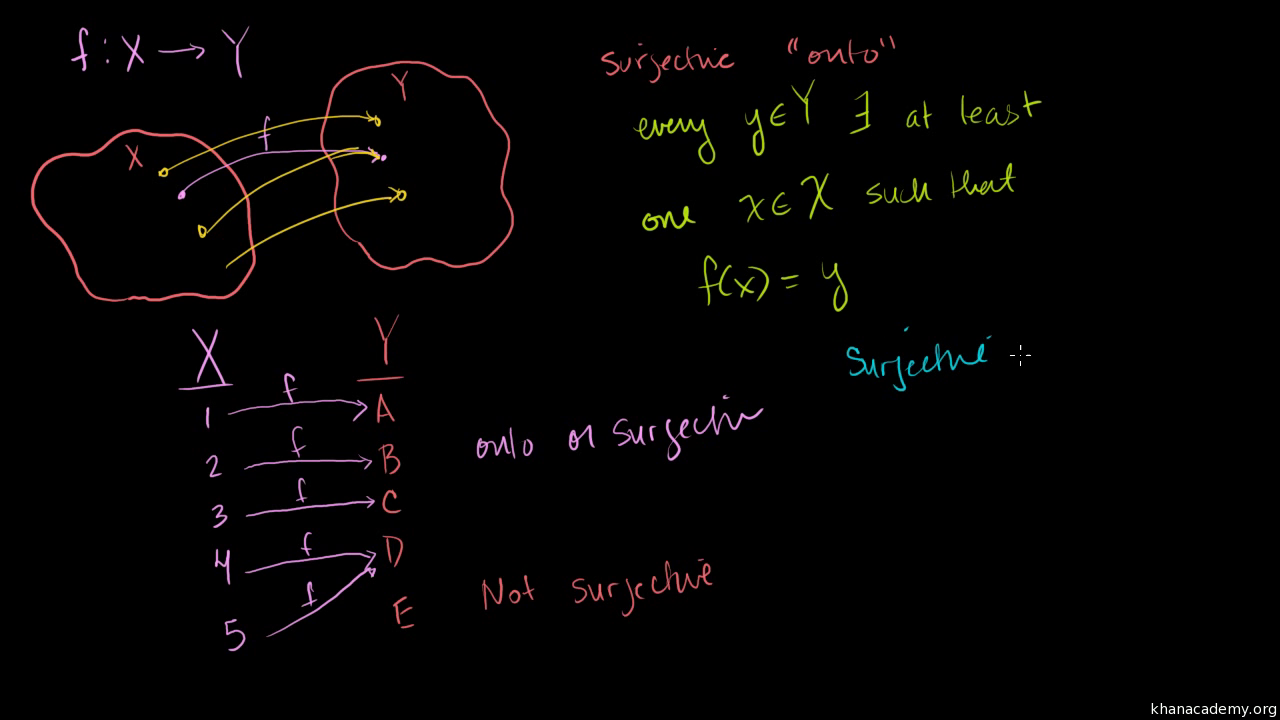



Surjective Onto And Injective One To One Functions Video Khan Academy




Discrete Mathematics Solutions To Homework 12 For Each Of The Following Sets Determine Whether 2 Is An Element Of That Set Pdf Free Download




Prove The Function F Z X Z Z Given By F M N 2m N Is Onto Surjective Youtube




If The Function F R 1 1 To A Definded By F X X 2 1 X 2 Is Surjective Youtube




Ex 1 2 7 State Whether One One Onto Or Bijective Class 12




Sol Abrev Hoja3m1 Eng Ejercicios Mathematics I Tema 3 Worksheet Di Erentiation Studocu
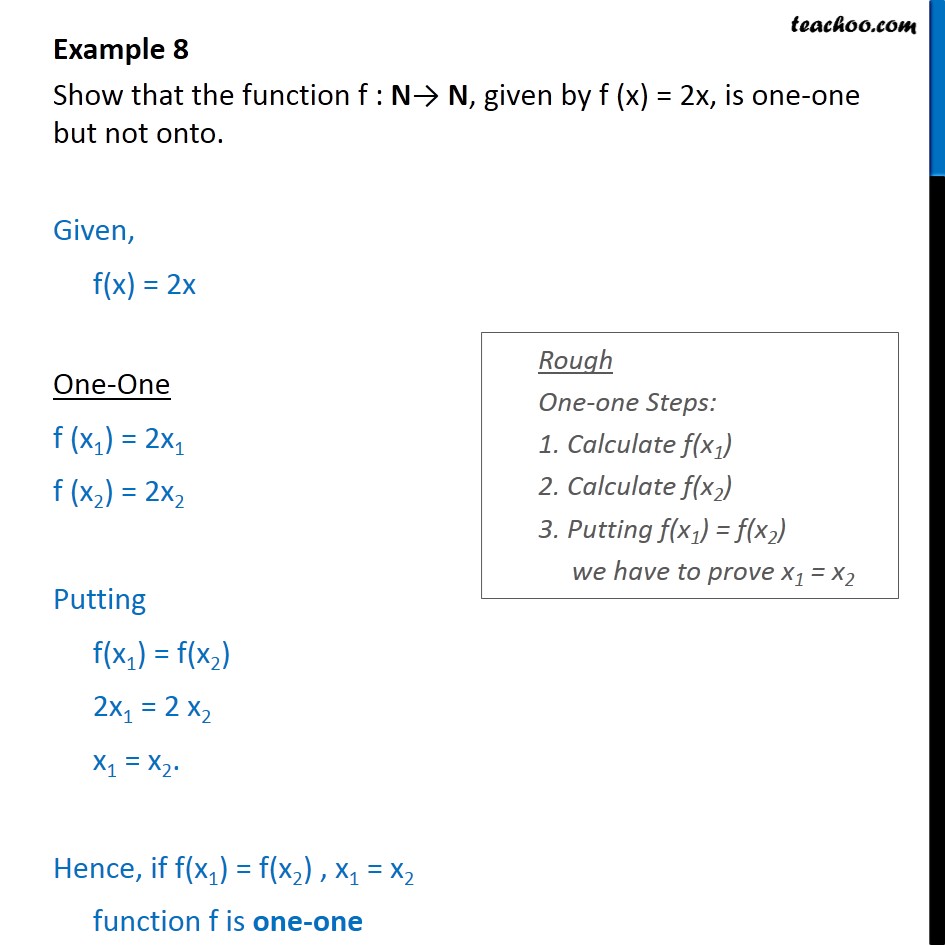



Example 8 Show F X 2x Is One One But Not Onto Examples
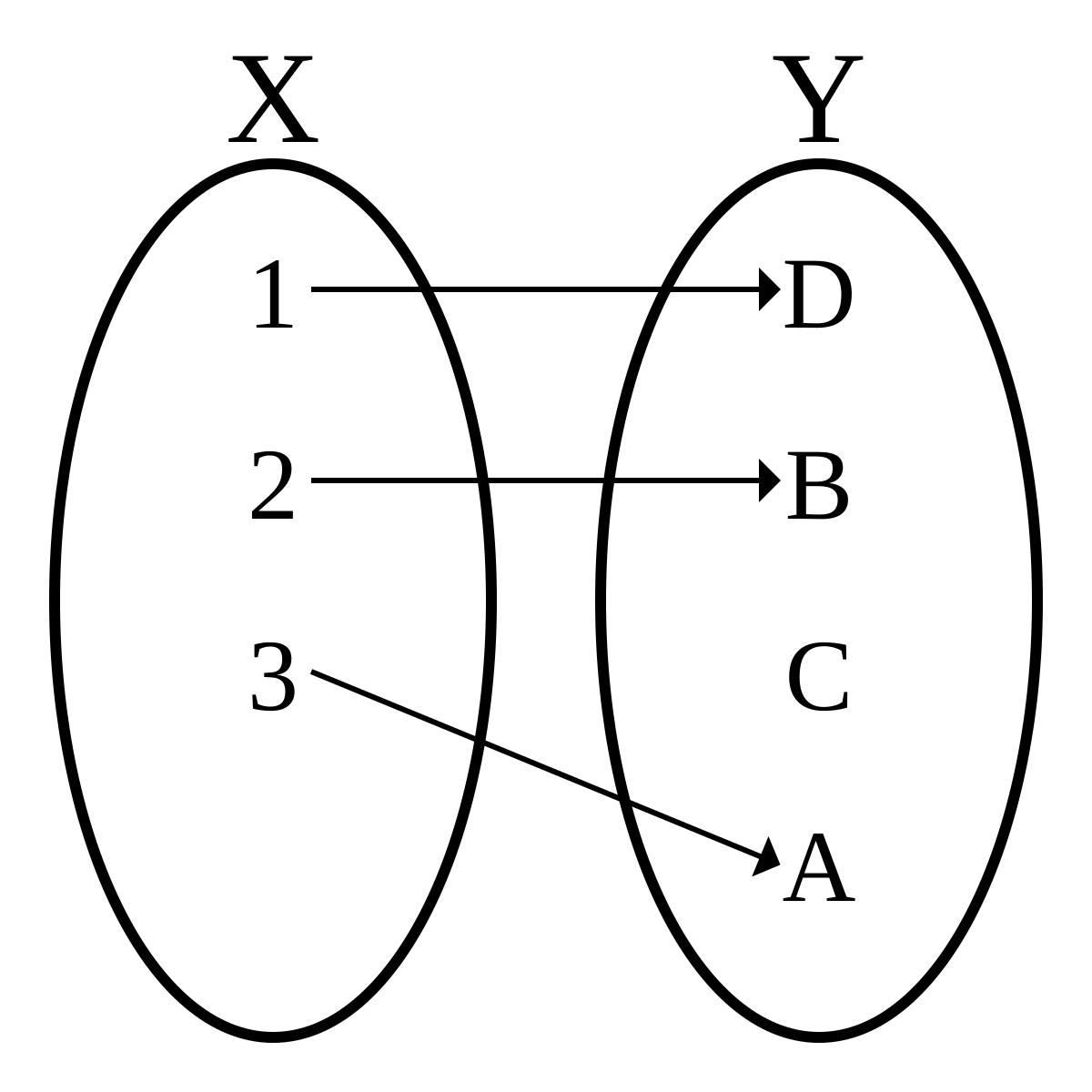



Injective Function Wikipedia
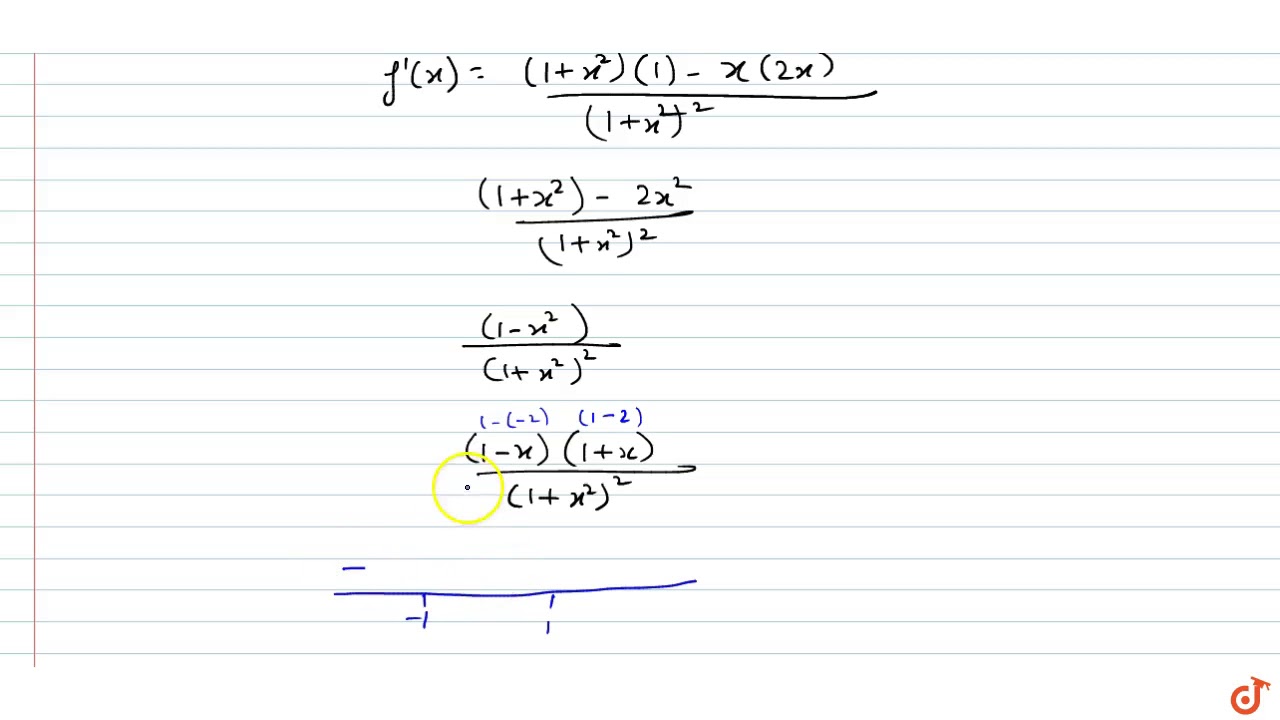



The Function F R Gt 1 2 1 2 Defined As F X X 1 X 2 Is Youtube
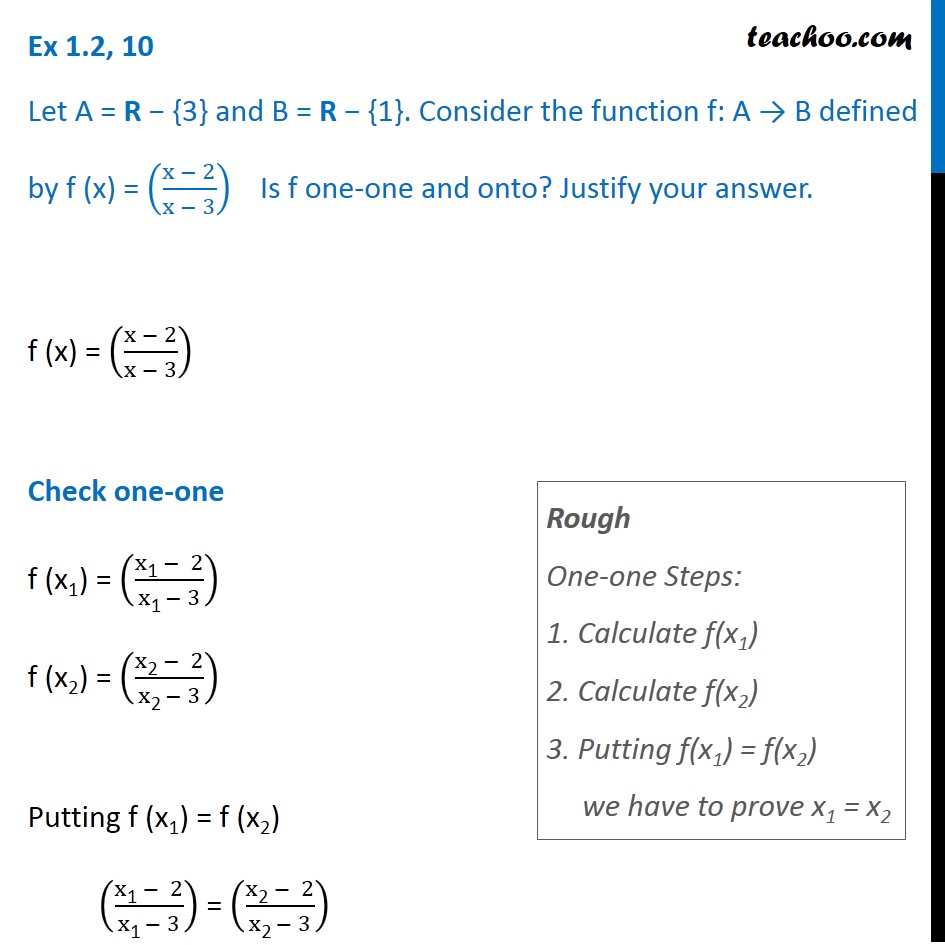



Ex 1 2 10 F X X 2 X 3 Is F One One Onto Class 12




Let F X X Root 1 X2 Show That Fofof X X Root 1 3x2 Brainly In




Bijection Function F X Y X Y 1 2x 2y 2 Mathematics Stack Exchange




Prove That The Function F X 3x 2 Is A Bijective Mapping Brainly In




Discrete Mathematics Solutions To Homework 12 For Each Of The Following Sets Determine Whether 2 Is An Element Of That Set Pdf Free Download



How To Prove The Rational Function F X 1 X 2 Is Surjective Onto Using The Definition Youtube




Solved Prove That The Function F R Rightarrow R Given B Chegg Com




Rd Sharma Solutions For Class 12 Maths Chapter 2 Function Download Free Pdf



Today S Topics Proof That A Given Function Is Injective Or Surjective Composite Functions Properties Of The Composite Functions Inverse Functions Proofs Ppt Download




Injections A Function Is One To One Injective If Each Element Of The Range Is The Image Of At Most One Element Of The Domain To Obtain A Precise Statement Ppt Download
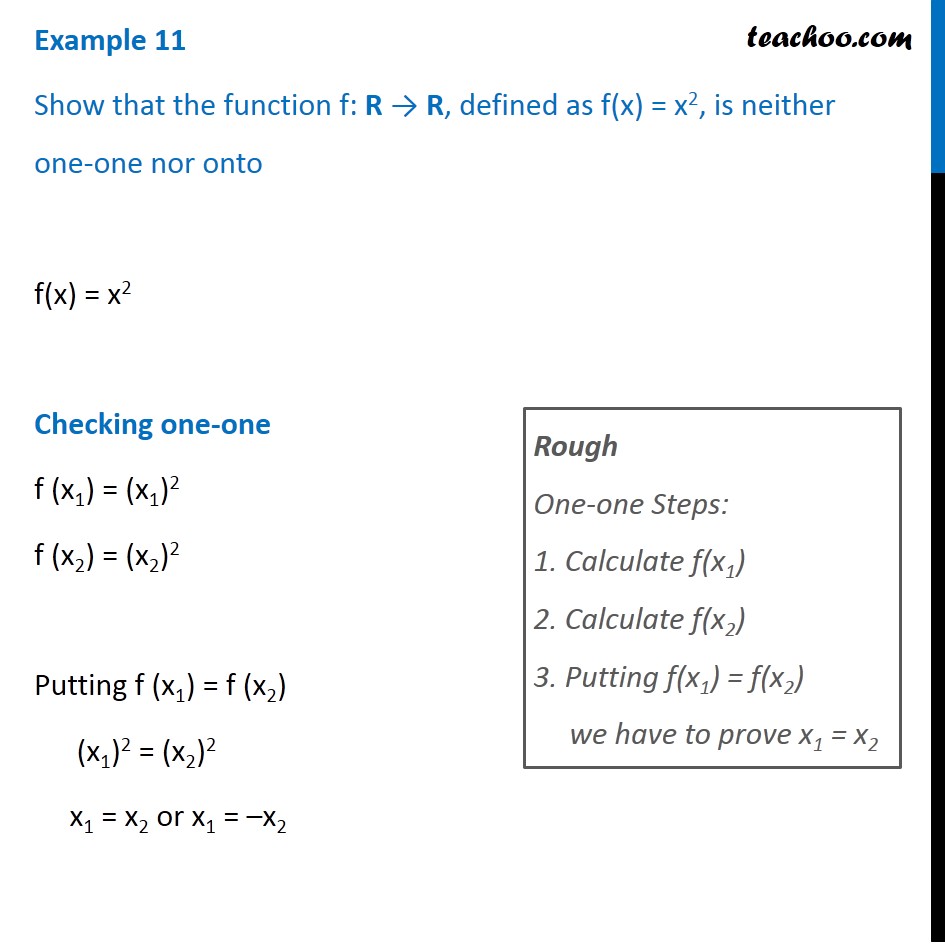



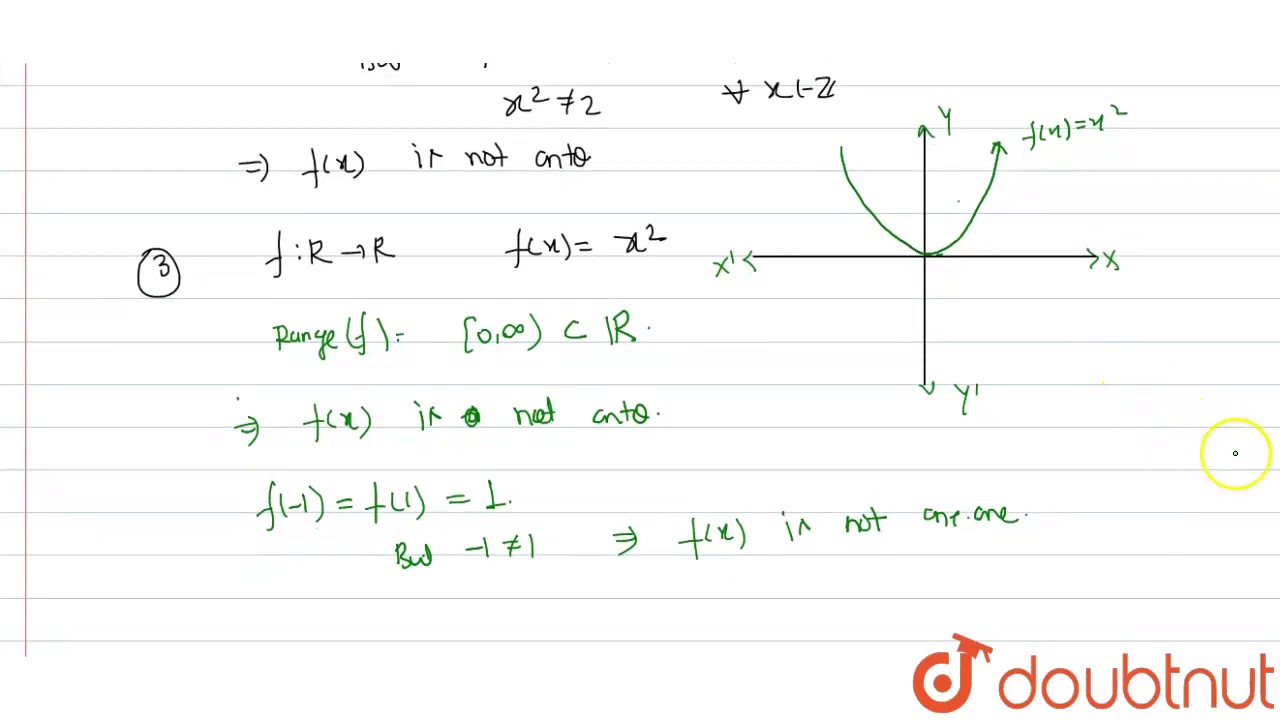
Example 11 Show F X X2 Is Neither One One Nor Onto Examples




Example 11 Show F X X2 Is Neither One One Nor Onto Examples
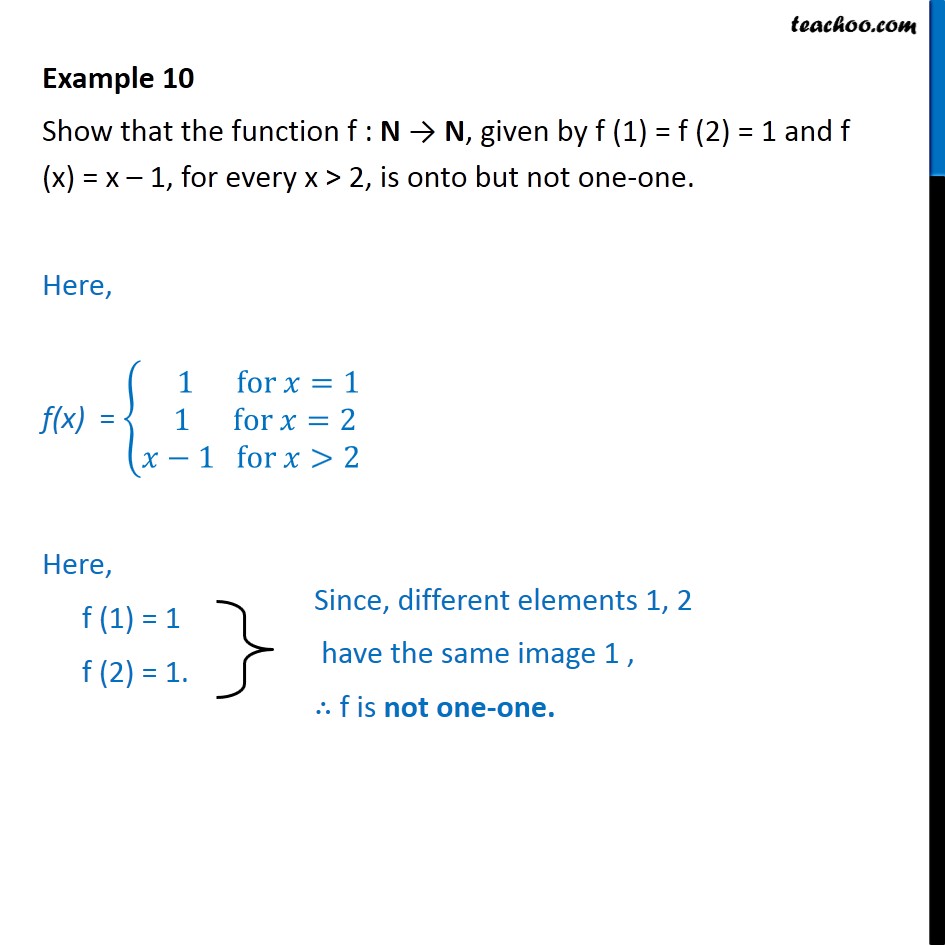



Example 10 Show F 1 F 2 1 And F X X 1 Is Onto




This Function Is Well U That This Function Is Chegg Com
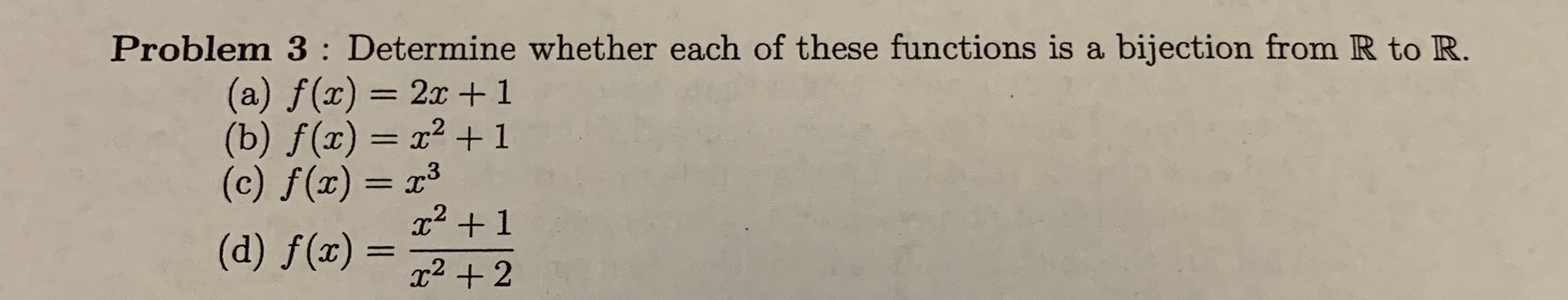



Solved Problem 3 Determine Whether Functions Bijection R R F X 2x 1 B F X X2 1 C F X X3 D F X X2 Q




Vatrix Match Type Question Column I 12 F R R Defined By F X X 113 F




F Pi2 Pi2 1 1 F X Sin X Is




In Each Of The Following Cases State Whether The Function Is One




State Whether The Function Is One One Onto Or Bijective F R R Defined By F X 1 X2 Brainly In




Bijective Function Simple English Wikipedia The Free Encyclopedia
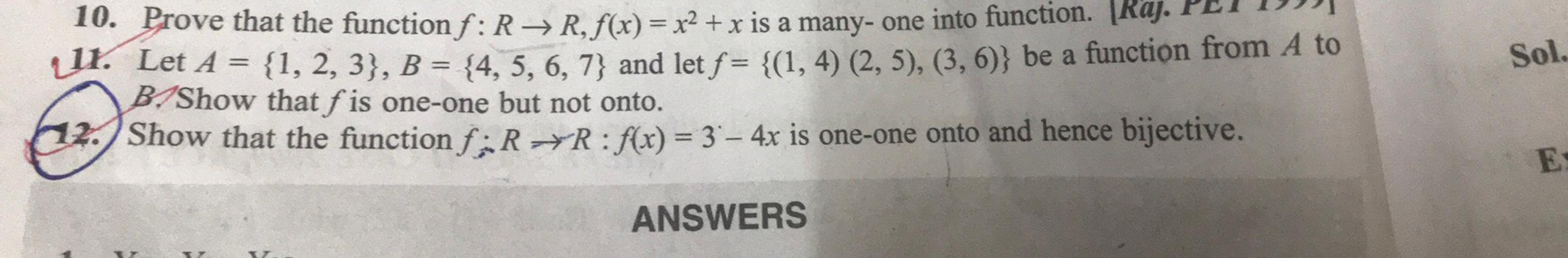



Sol 10 Prove That The Function F Rr F X X2 X Is Math




Q 16 Classify The Following Functions F X Defined In Rrarrr As Injective Surjective Both O Youtube




The Function F R R F X X 2 Is A Injective But Not Surjectiv
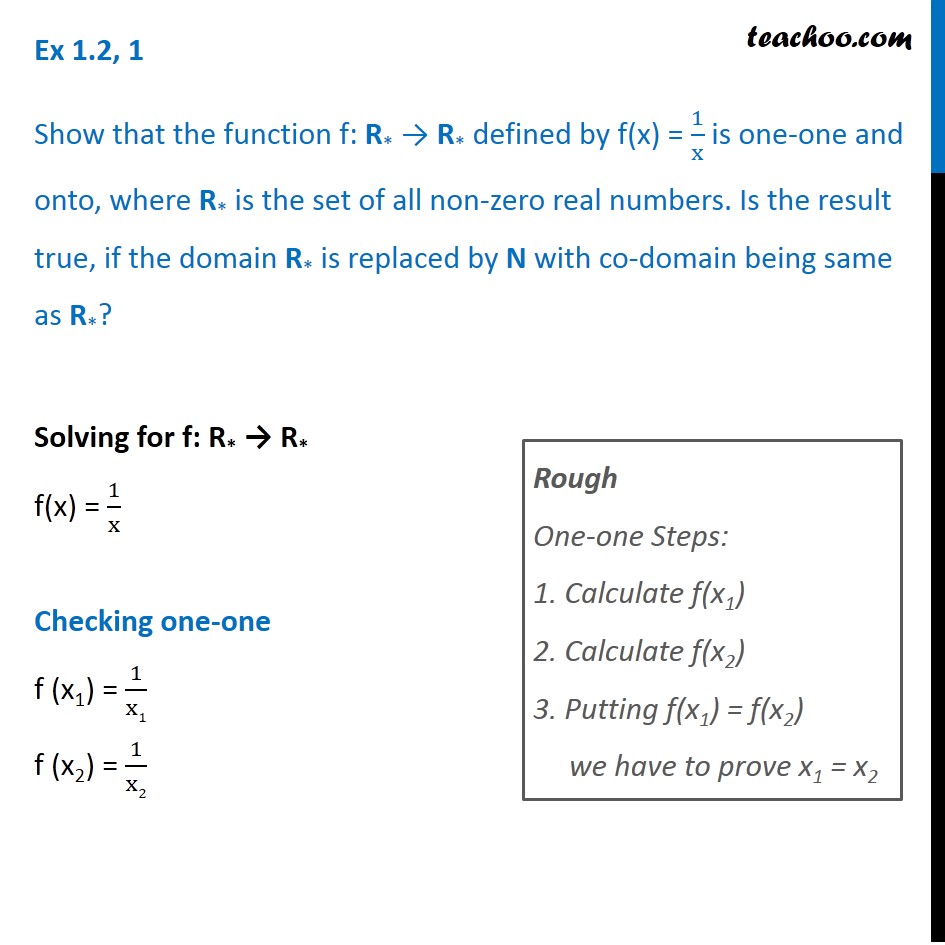



Ex 1 2 1 Class 12 Maths Show F X 1 X Is One One Onto Where R




Classify F R R Defined By F X X X 2 1 As Injection




Notes On Topics Of Algebra Notes




The Function F Rvec 1 2 1 2 Defined As F X X 1 X 2 Is




Problem 1 Determine Whether Each Of These Functions Chegg Com




Answered 1 Let F R R Be Defined By F X 2x Bartleby




Show That F R R Defined As F X X 3 Is Bijection Maths Relations And Functions Meritnation Com




Let A X Xepsilonr F Is Defined From Ararrr As F X 2x X
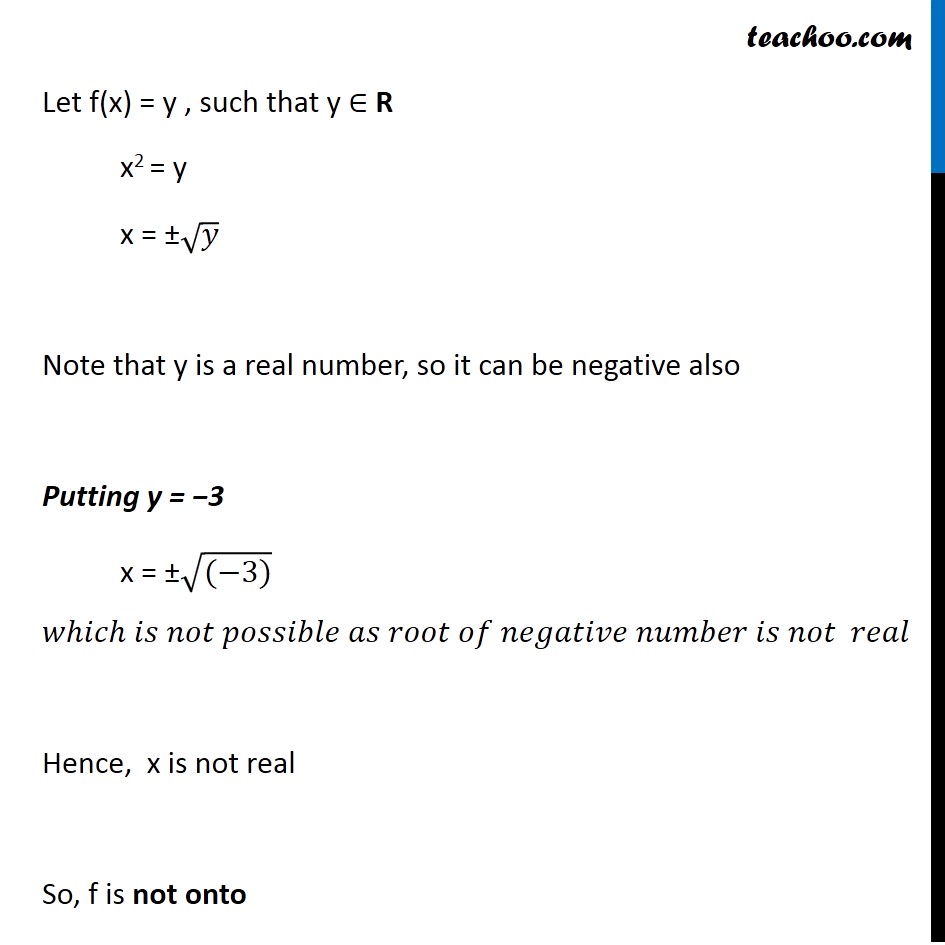



Example 11 Show F X X2 Is Neither One One Nor Onto Examples




Help Maths




Ex 1 2 2 Check Injectivity And Surjectivity Of I F X X 2



No comments:
Post a Comment