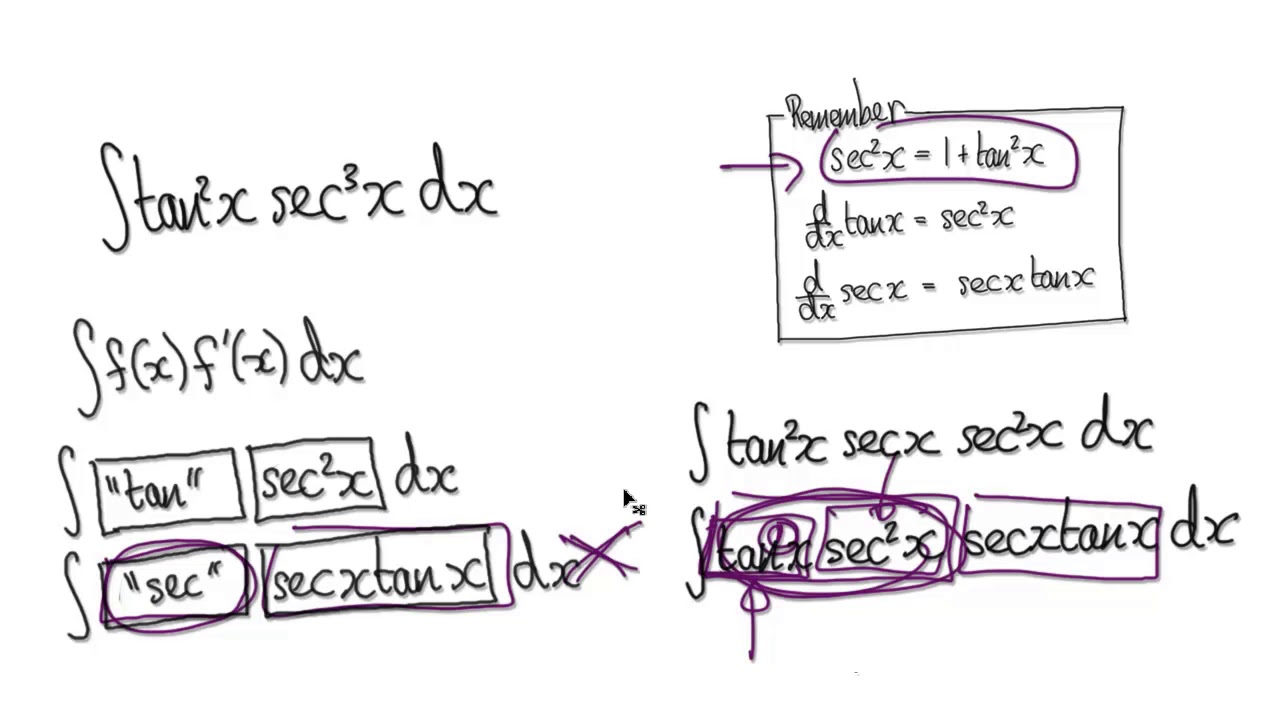
Use the identities sec^2 (x) = tan^2 (x) 1 and csc^2 (x) = 1 cot^2 (x), both are derived from the pythagorean identity of 1 = sin^2 (x) cos^2 (x) by dividing through by either sin 2 or cos 2 2 View Entire Discussion (1 Comments) Abhishek K LH S = sec2x csc2x = 1 cos2x 1 sin2x = sin2x cos2x sin2x ⋅ cos2x = 1 cos2x ⋅ csc2x = sec2x ⋅ csc2x = RH S Answer link

Tan 2x Cot 2x 2 Sec 2x Csc 2x Brainly In
Tan^2x-sec^2x 2=cosec^2x-cot^2x
Tan^2x-sec^2x 2=cosec^2x-cot^2x-




Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco



Www Thestudentroom Co Uk Attachment Php Attachmentid D



2




Calderglen Maths For Our Wonderful New Advanced Higher Pupils Here Are The Answers So Far To The Portfolio Questions On Which You Have Been Working T Co Himarma0lj



Tan2x Sec2x ただの悪魔の画像



1




Rd Sharma Solutions For Class 11 Chapter 11 Trigonometric Equations Updated For 21 22 Coolgyan Org




Tan 2x Cot 2x 2
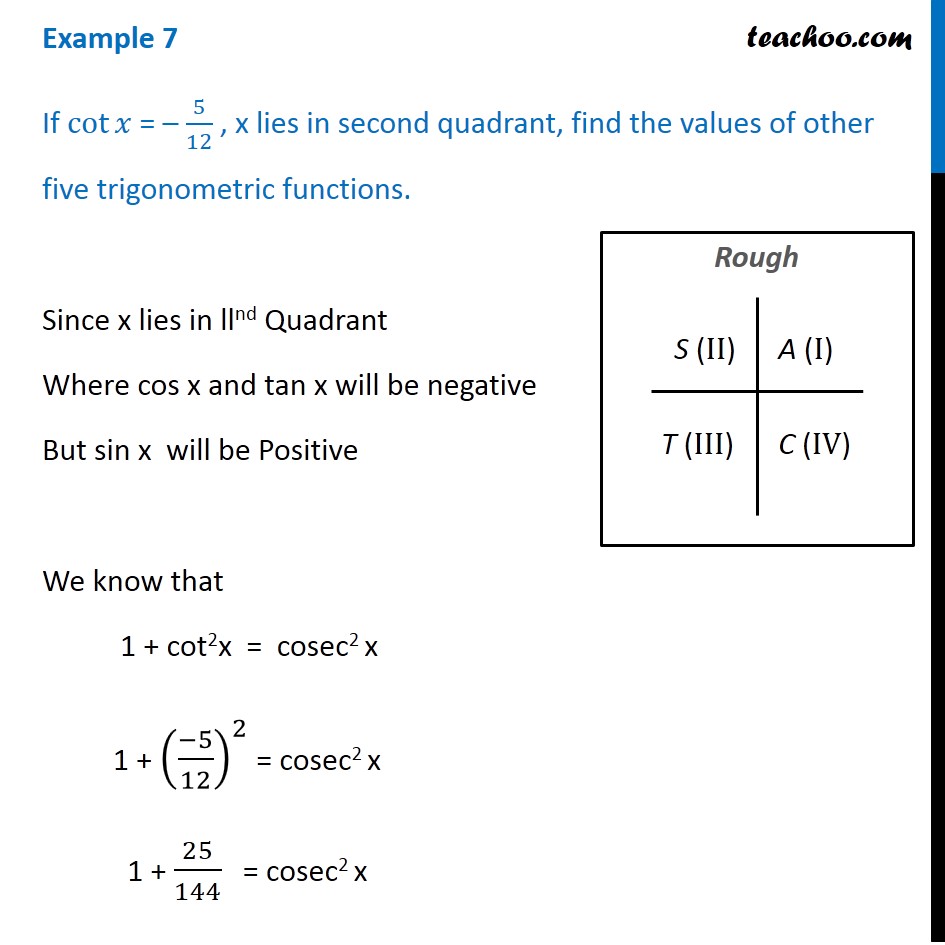



Example 7 If Cot X 5 12 X Lies In Second Quadrant



2




Tanx Cotx 2 Sec 2x Cosec 2x Brainly In




Help Pls Thankyou Ask Manytutors




Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco



Http Www Mpsaz Org Mtnview Staff Clpavlovic Collegealgebra Resources Files Assignment 6 3 Day 1 3 Worksheet Key Pdf



1




Sec 2 X Cosec 2x Tan X Cot X Prove It Brainly In




10 Trigonometry Trigonometric Functions Sine



Www Jethwamaths Com Uploads 1 3 3 1 Year 2 Trig Compilation Pdf Pdf



2




Prove That 2sec 2x Sec 4x 2cosec 2x Cosec 4x 1 Tan 8x Tan 4x Youtube
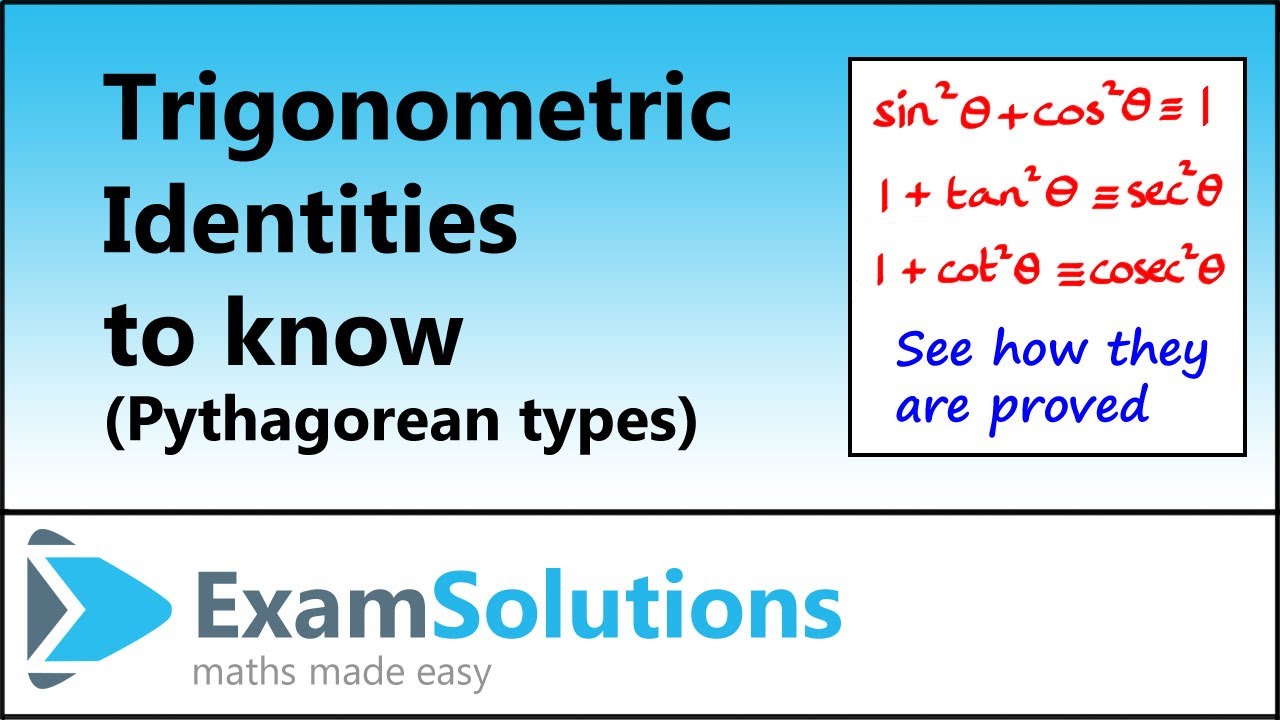



Sin2x Cos2x 1 1 Tan2x Sec2x 1 Cot2x Cosec2x Revisely



1



10 A Show That Cosec 2x Cot 2x Cot X B Hence Solv Gauthmath



Solved Activity Exercise No 9 Differentiation Of Trigonometric Functions Find Y Of The Following Function Dx 1 Y Sin2 2x Cos 4x 2 Y Sec2 Course Hero



Sweet Refusal Excavation Cot X Square Root Of 8 4 Bluecheddarbrie Com



Solved Activity Exercise No 9 Differentiation Of Trigonometric Functions Find Y Of The Following Function Dx 1 Y Sin2 2x Cos 4x 2 Y Sec2 Course Hero



Http Www Ummg Gov Mm Media Documents 18integration Pdf




2 Sec 2a Sec 4a 2 Cosec 2a Cosec 4 Cot 4 A Tan 4a Maths Introduction To Trigonometry Meritnation Com



How To Prove The Identity In Tanx Cotx 2 Sin2x Quora




Trigonometry Reciprocal Identities Expii



8 T Tan 2x Cot 2 X 1dx 9 T Frac 1 Cos Gauthmath




Find The Value Of Sec 2x Cosec 2x Tan 2 Cot 2x X Belongs 0 90 X Not Equal To 45 Brainly In




Rd Sharma Solutions For Class 11 Maths Updated 21 22 Chapter 11 Trigonometric Equations Download Free Pdf



Http Www Jkshahclasses Com Images Fyjc Maths 18 19 Ch 5 trigonometric fns 5 1 5 2 Pdf
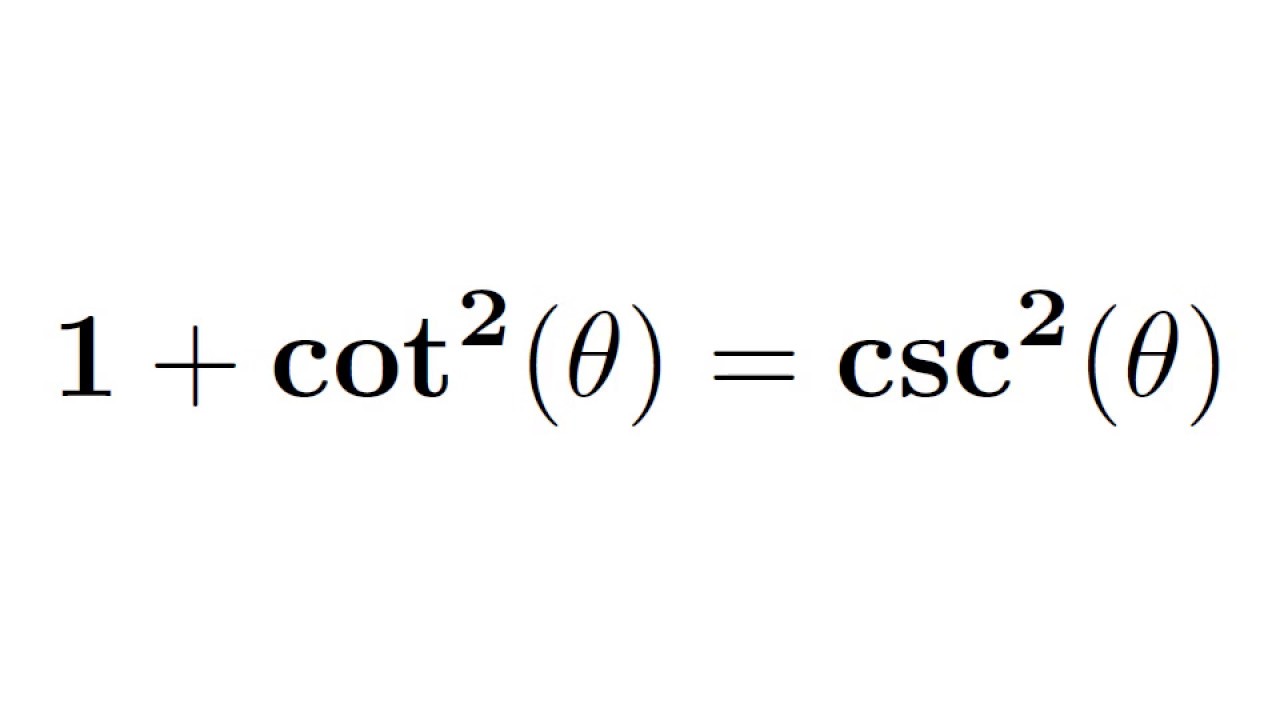



Paletă Inspector Ieșire 1 Cot 2x Cosec 2x Montrealtrainersgroup Com




Solve Tan 2x Cot 2x Sec 2x Sin 2x Cos 2x Cosec 2x Is Brainly In




1 Sin 2x Tan 2x Sec Sec 2 X Csc 2x 1 1 C Gauthmath



Solved K U 1 Differentiate The Following Choose 4 Only Marks A Y Tan 2x 1 Cot 2x B F X Xsin X 1 X C G X Sec Quot 6x Cos Course Hero




Paletă Inspector Ieșire 1 Cot 2x Cosec 2x Montrealtrainersgroup Com




Cosec 2x Shefalitayal




Nda Paper Solved Pdf Pdf Txt




Trigonometry Questions For All Competitive Examinations




Integration Calculus Notes




Tan 2 X Tan 2 X 1 Cosec 2 X Sec 2 X Cosec 2 X 1 Sin 2 X Cos 2 X Brainly In
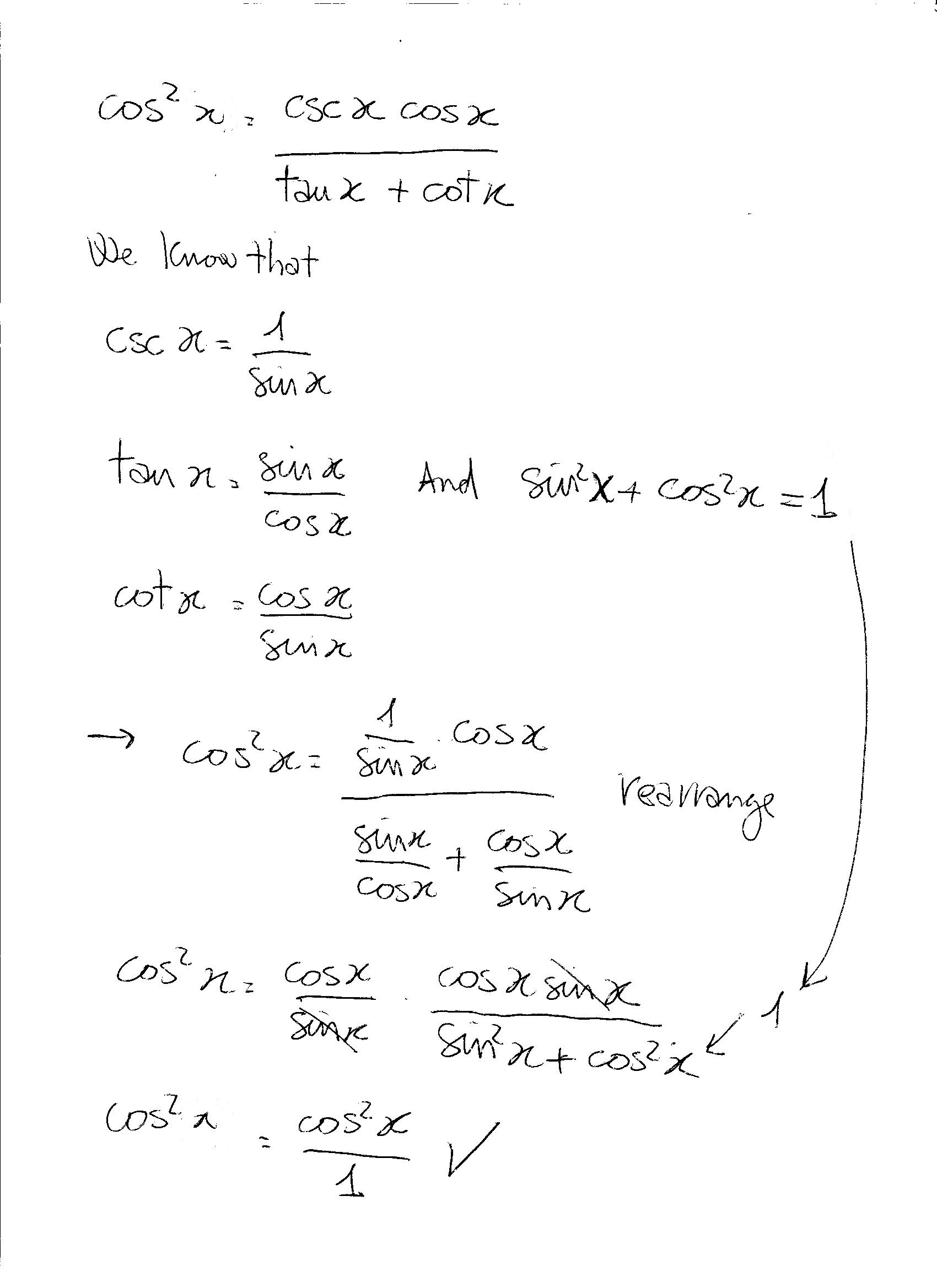



How Do You Prove Cos 2x Cscx Cosx Tanx Cotx Socratic




Integration Calculus Notes




How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic



2




Integration Trig Identities Ppt Download
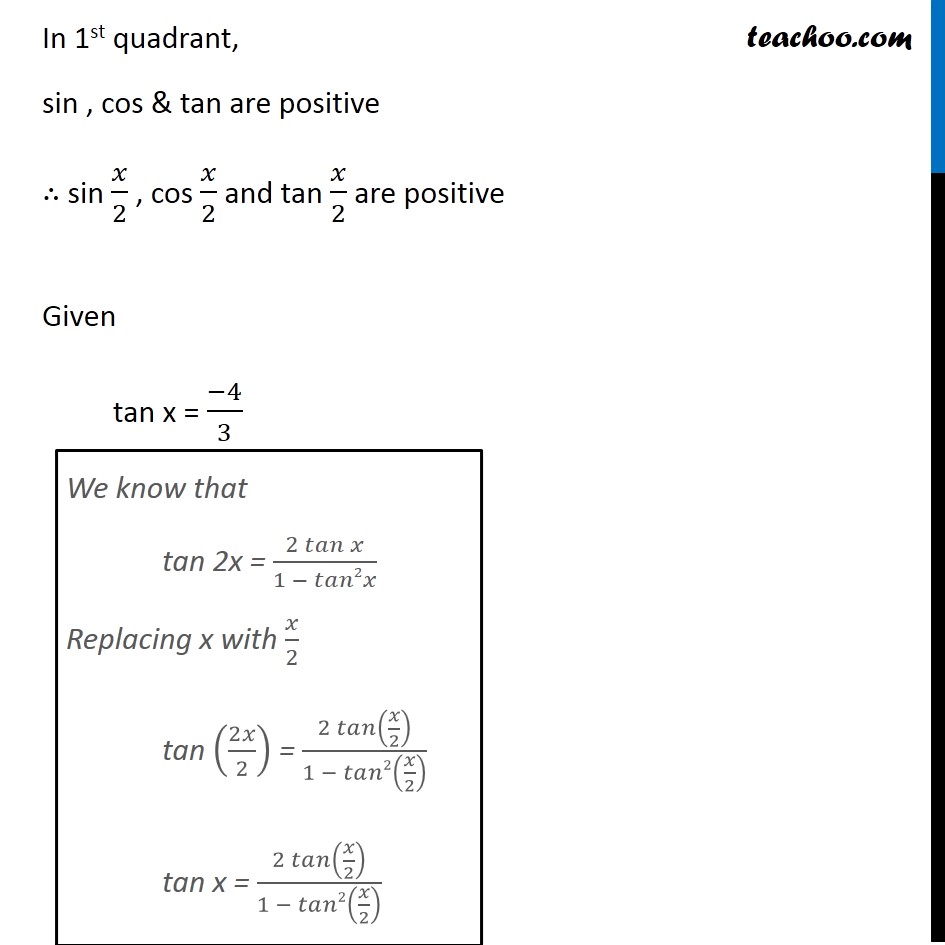



Misc 8 Tan X 4 3 Find Sin X 2 Cos X 2 And Tan X 2



2




1 Question 7 I Find S X 6x2 Dx Giving The Chegg Com




Integral Of Tan 2x Secx




Derivatives Of Tan X And Cot X Video Khan Academy




How Many Can You Derive From First Principles Ppt Download




Prove That Sec 2x Cosec 2x Tan 2x Cot 2x




Using Trigonometric Identities Video Khan Academy



Http Www Math Brown Edu Tomg Formulas Pdf
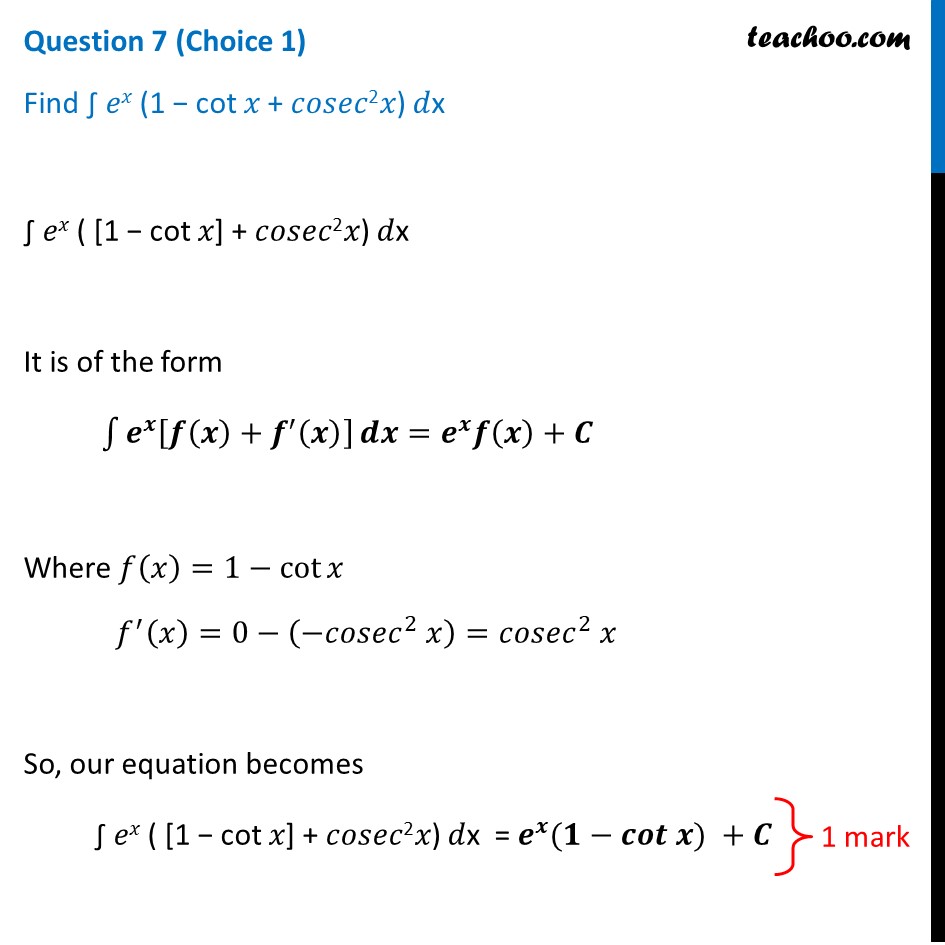



Find E X 1 Cot X Cosec 2 X Dx Integration Video Teachoo



2




Reciprocal Trig Functions Ppt Download



2




Tan 2x Cot 2x 2 Youtube




How Do You Integrate Csc2x Cot2x 2 Dx Socratic



2




Powers Of Trigonometric Functions




How Many Can You Derive From First Principles Ppt Download



Mathsmartinthomas Files Wordpress Com 15 12 C3 Trig Past Paper Q Markscheme Pdf




Sweet Refusal Excavation Cot X Square Root Of 8 4 Bluecheddarbrie Com
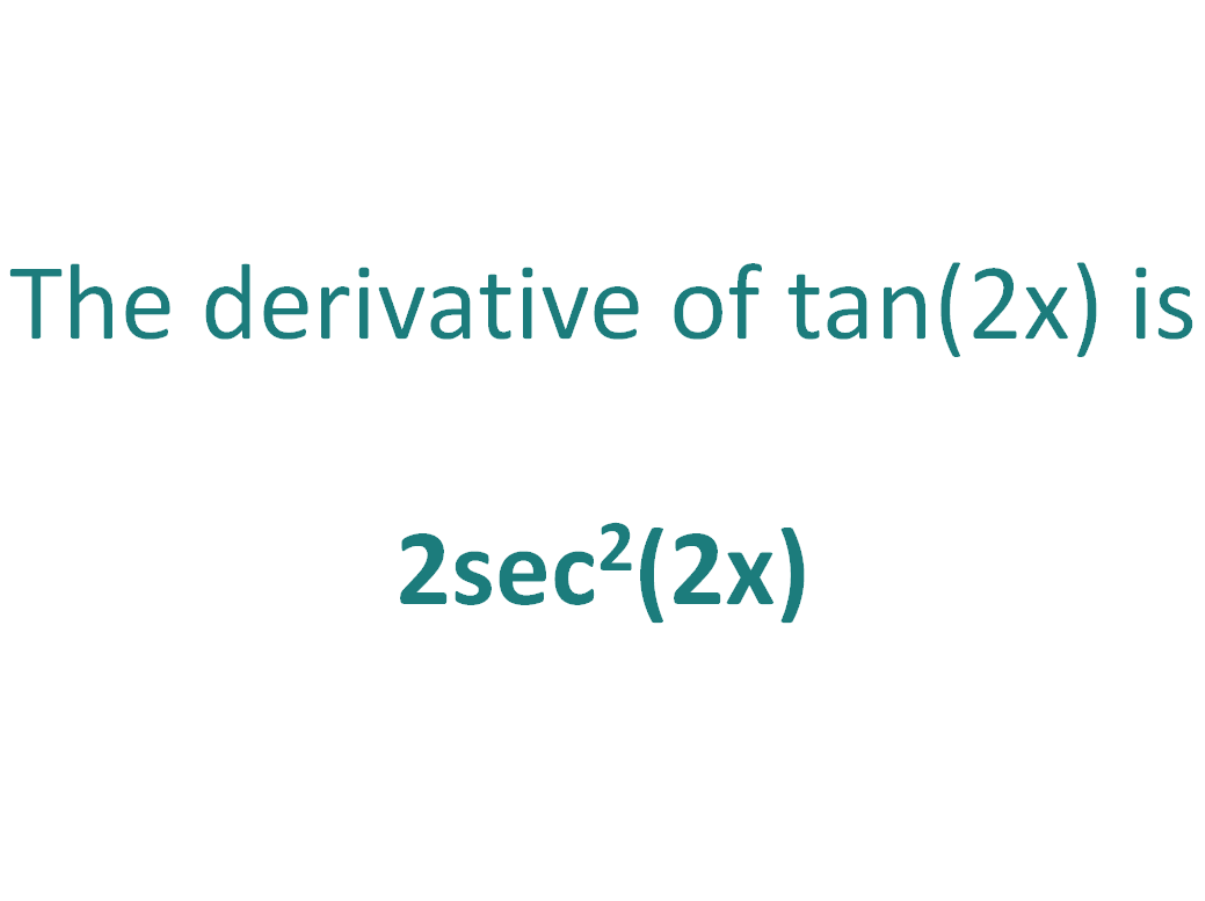



Trigonometric Functions Derivativeit




Integration Calculus Notes




Trigonometry Questions For All Competitive Examinations




Integration Calculus Notes



2



Www Jstor Org Stable




Tan 2x Cot 2x 2 Sec 2x Csc 2x Brainly In



Pmt Physicsandmathstutor Com Download Maths A Level C3 Topic Qs Edexcel Set 1 C3 trigonometry trigonometric identities Pdf




If F X Log Sec 2 X Cot 2x For e0 And K For X 0 Is Continuous At X 0 Then K Is




Consider The Following Equations 1 Cosec 2x Sec 2x Cosec 2xsec 2x 2 Sec 2x Tan 2x Sec 2xtan 2x 3 Cosec 2x Tan 2x Cot 2x



2



Reciprocal Trig Functions Ppt Download




Number Of Solutions Of The Equation 4sin 2x Tan 2x Cot 2x 2 6 In 0 Pi Is




67 Knowledge Gateway Ideas In 21 Knowledge Gateway Math Equations




Tan2x Sec2x ただの悪魔の画像




Exercise 7 3 Integrals Of The Functions In Exercises 1 To 22 2 Sin 3x Cos 4x 3 Cos 2x Cos 4x Cos 6x 5 Sin X Cos 6 Sin X Sin 2x




Derive 1 Tan 2x Sec 2x And 1 Cot 2x Text Cosec 2x Geometrically Mathematics Stack Exchange




How Many Can You Derive From First Principles Ppt Download




Prove That Sec 2x Cosec 2x Tan 2x Cot 2x



2




Limit Trigonometric Function 2 Sec 2x 1 Tan X Youtube



2
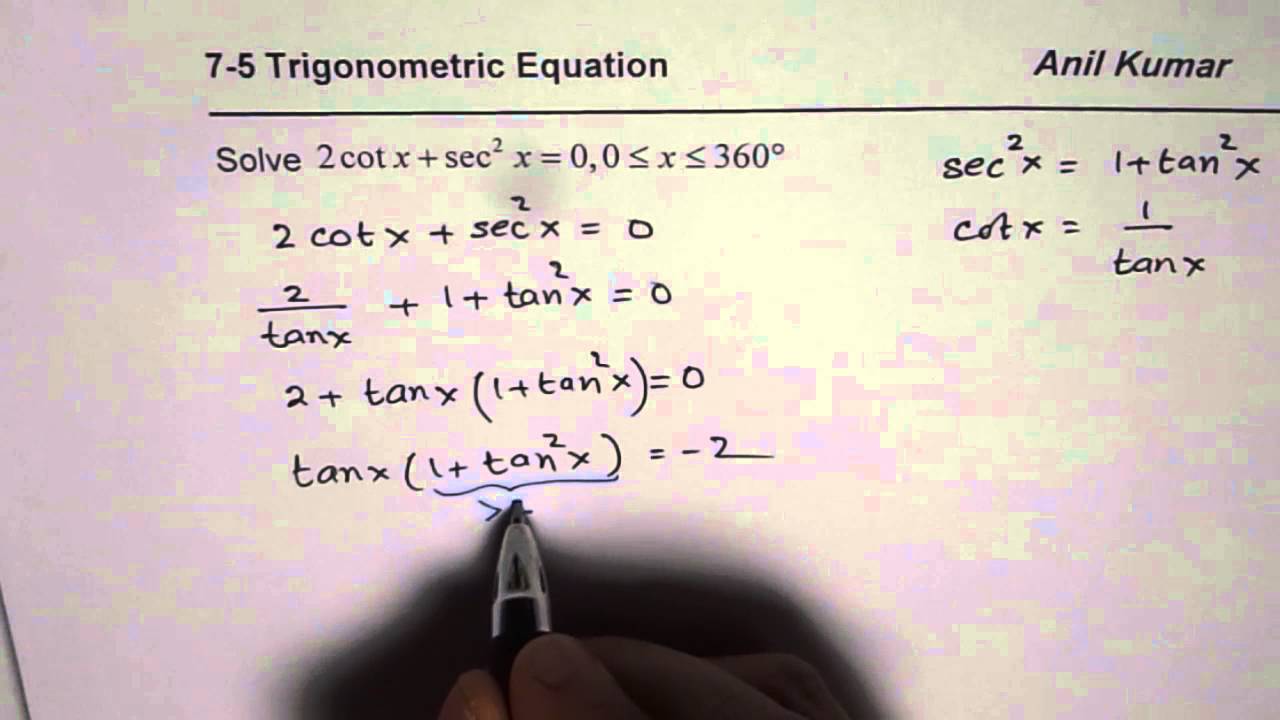



Cot X Plus Sec 2x Equal 2 Trigonometric Equation Solution Youtube



How To Show That Sec X Tan X 1 Sec X Tan X Quora




Find The General Solution Of Cot X Tan X 2 Cosec X Youtube




Integral Of Tan 2x Secx




Worksheet On Trigonometry Review With Answer Key Docsity



Cosec 2x Shefalitayal




Tan 2x Cot 2x 2 Sec 2 Cosec 2pls Give The Answer It S Extremely Urgent Pls Pls Brainly In
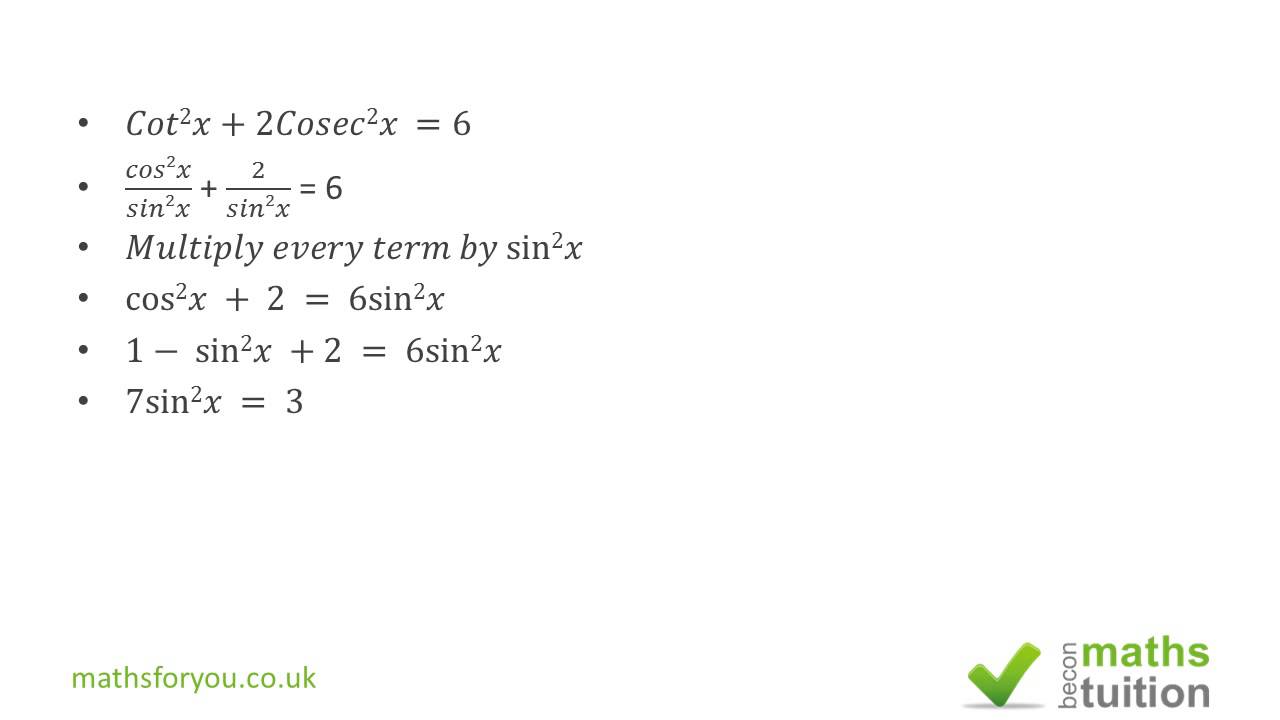



Solving Cot 2x 2cosec 2x 6 For Values 0 X 360 Part 2 Youtube



Http Www Ppup Ac In Download Econtent Pdf B sc Math part1 paper 2 Pdf




Ge5 Frac Sec X In Xtarctan X Cotx Sin 2x D Cosec Gauthmath



1
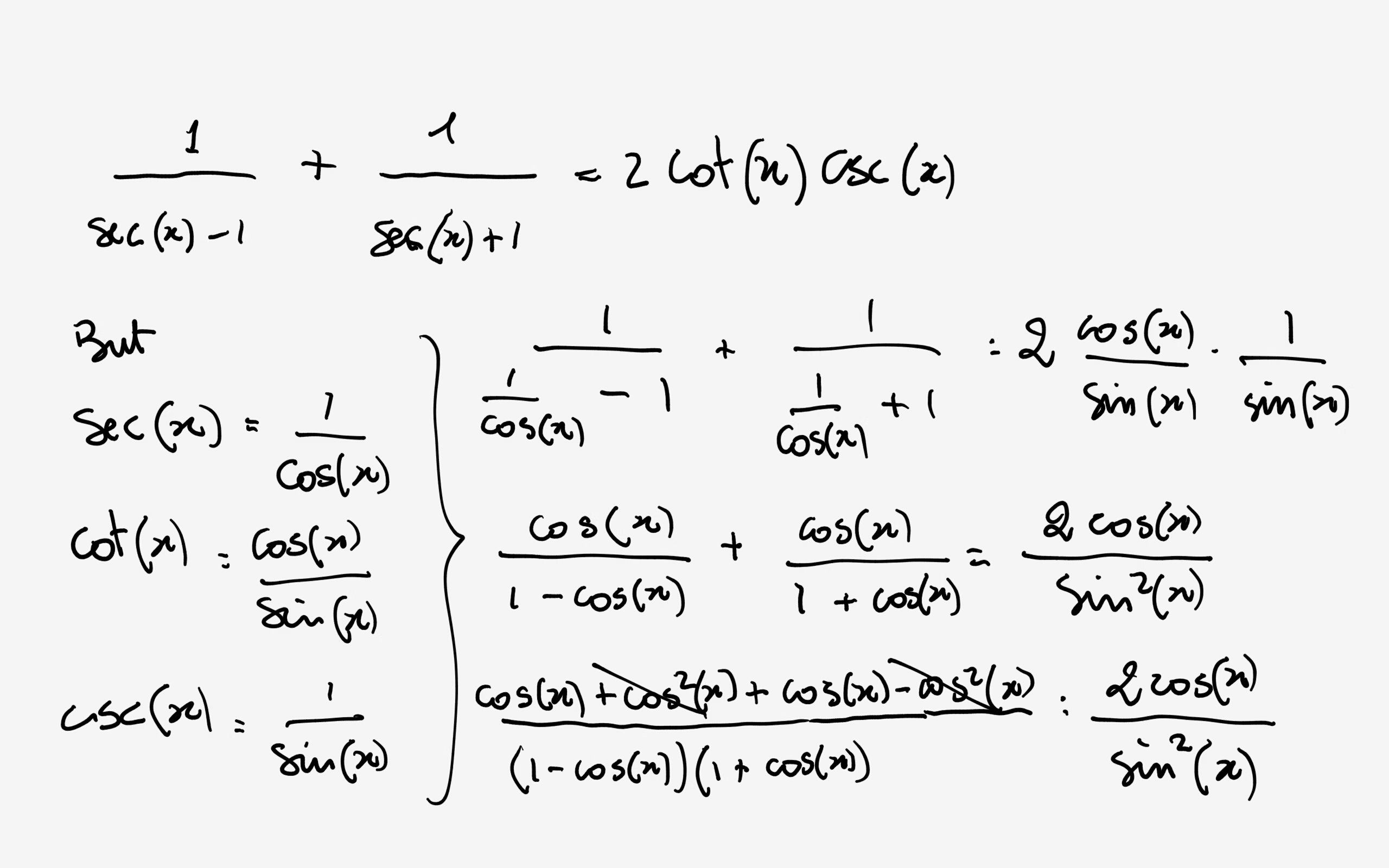



How Do You Verify 1 Secx 1 1 Secx 1 2cotxcscx Socratic




Answered Find The Derivatives Of The Following Bartleby




Xxi If 2y Cos 8 Xsin 8 And 2x Sec 8 Y Cosec 8 3 Then What Maths Trigonometry Meritnation Com



No comments:
Post a Comment